“No, you are almost certainly not going to be hit by a 10-story, 23-ton piece of a rocket hurtling back to Earth. That said, the chances are not zero.” Thus The New York Times on May 6 writing about the impending reentry of the first stage of the Chinese CZ-5B rocket, which had been launched the day before to test a spacecraft prototype. Unlike most rockets sent into orbit these days, which are designed to either burn up in the atmosphere or land in the ocean, this rocket stage would experience an “uncontrolled re-entry” and crash in an unknown location.
Though the article, like others about the rocket, emphasized the remote chance that the reader would be struck by the rocket, it didn’t provide much in the way of specifics. Given the sheer size of the rocket, one would be forgiven for wondering—just how likely is it that this rocket hits me, anyway? As a statistician, it’s impossible not to ask this question.
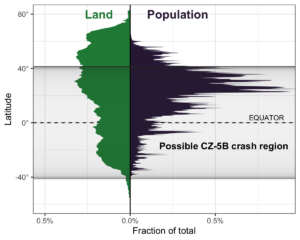
Because of the angle of the rocket’s orbit, it had to come down somewhere between latitudes 41.5° S and 41.5° N. But since it was traveling at 18,000 miles per hour (29,000 km/h), where in this huge swath of the planet’s surface it would fall was anyone’s guess. Concernedly, this section of the Earth contains most of its land mass and all but one billion of the world’s population, as the diagram here shows.
To get an actual probability, we compare the area occupied by people to the total area in which the rocket could come down. If we assume that the center of the debris field being within 10 meters of a person would cause them serious harm, we can calculate that there was roughly a 0.66%, or 1 in 151, chance that anyone on Earth would be struck by a piece of debris. Not huge, certainly, but still rather high, considering that the chance could have been zero had the rocket been deliberately designed to fall into the ocean.
But a 1 in 151 chance that anyone was hit translates to only a 1 in a trillion chance that you, specifically, were a victim. In comparison, the chance that you are struck by lightning this year is “only” 1 in 700,000—more than a billion times more likely.
So at first glance it may seem foolish to focus at all on such an unlikely event. And ultimately the rocket did fall harmlessly into the Indian Ocean in the early hours of May 9. But these kinds of back-of-the-envelope calculations obscure other considerations which may explain the intense interest this event received.

First, there’s the type of uncertainty. While most of us consider something like a lightning strike to be truly random, the uncertainty about where CZ-5B would fall was of a slightly different sort—we knew it had to come down somewhere, and it seemed possible in principle to know exactly where, if one had enough information. We just weren’t in a position to know; as far as we were concerned, we could treat the rocket’s fall like a lightning strike. This dichotomy—between “truly” random events and those for which we are merely missing key details—is a common one, and the boundary between the two may shift over time. Before humanity had an accurate model of the solar system, astronomical events like eclipses happened seemingly at random, and were consequently ascribed religious significance. Perhaps some day we will have good enough weather data and models to predict the location of specific lightning strikes.
Second, the way in which the 1-in-151 risk was distributed across the global population probably made a difference in how the crash was perceived. Based on the way the rocket orbited, people near the latitudinal extremes of 41.5° were at moderately higher risk than those near the equator. But other than this, the risk was relatively evenly distributed across the 6.8 billion people in the crash area. The median risk of being struck—that for which half of people have a higher risk and half have a lower risk—was just about the same as the risk for a randomly chosen person, at 1 in 1.2 trillion. In contrast, most other disasters are concentrated in a much smaller region of the globe. In these cases, the median risk is much, much lower, since the risk is borne only by a small fraction of the global population.
Other factors—the size of the rocket, the rarity of uncontrolled re-entries, and geopolitical tensions—contributed to the newsworthiness of the event. But it’s important to remember that both the cause and the distribution of uncertainty over the population matter when evaluating rare events and understanding how they will be perceived by the world—not just the total probability.